ABSTRACT
Information about the forces acting on cylindrical structures subjected to wave impact is of significant importance in ocean engineering and naval architecture. The design of structures that must survive in a wave environment depends on knowledge of the forces that occur at impact. Impact loads due to wave slamming on horizontal members of an offshore structure are of considerable interest in the context of offshore design, particularly because such loads can give rise to structural failure. Predictions of the wave slamming force generally involve the use of a slamming coefficient. The purpose of this thesis is to analyse the wave impact forces acting on a fixed, slender, horizontal circular cylinder in the vicinity of the free surface, taking account of the intermittent submergence and wave slamming. It presents a new mathematical model for the impact forces acting on a horizontal circular cylinder from the instant of impact to full immersion. Two new expressions are derived for the slamming coefficient, the first expression as a function of the Froude number and the second expression as a function of the Keulegan-Carpenter number. A computer program is developed on the basis of the aforesaid theoretical analysis. The program is written in Fortran 90/95 and executed on the personal computer. The computational results are plotted to show the variation of the slamming coefficient with the Froude number, Keulegan-Carpenter number, wave amplitude, cylinder diameter, depth of cylinder immersion, instantaneous height of the wave surface above the mean water level and the added mass per unit length of the cylinder. In order to check the validity of the mathematical model developed, the present results are compared with the theoretical and experimental results of other investigators.
Introduction
Hydrodynamic impact may be defined in general fashion as the forcible, sudden contact of a body, or any part thereof, with the surface of water. A fast planing craft, running through and into waves, suffers impact loading when a portion of the moving bottom surface meets the advancing side of a wave, practically parallel to it. The vertical forces and changes in acceleration on a small, fast, planing boat are frequently so large as to cause it to jump violently up and down. Impact also occurs when there is sudden contact of a liquid surface with a body or a ship; in other words, when the liquid is moving rather than the ship. Large waves often strike sea walls with impact, and breaking wave’s impact upon ships which are not in motion.
Interest in this field of hydrodynamics arose more than a half century ago in connection with the problem of the landing of flying boats and seaplanes. Increases in the takeoff and landing speeds of flying boats and seaplanes, and in the running speeds of racing motorboats, coupled with the use of these crafts in rougher water, brought with them the need to estimate or to predict the water-impact forces and pressures on their bottoms. The latter were almost always wedge-shaped in section. Instrumentation was not then available to record these rapidly applied loads and strains, so the problem was attacked analytically (Szebehely, 1959).
The wave impact is generally defined as the early stages of the penetration of a solid body into a wave surface. At the instant of contact the fluid in the vicinity of the body undergoes large accelerations which give rise to large forces. As the body becomes more fully immersed, forces due to viscous drag and separation effects become predominant. Thus the inertia and drag coefficients used in the classical Morison equation (Morison et al., 1950) are not constant, and the problem becomes very difficult, even for simple geometries.
When a body enters a free water surface at speed it experiences large transient forces due to the acceleration field it sets up within the water. The forces are generally termed impact or slamming loads and are often of considerable practical importance. Offshore structures often have appreciable numbers of main structural and bracing members near the still water level. As waves pass the structure some of these members are alternately exposed to the air and then submerged in the water. Each time they enter the water there are slamming forces. Failures of some members have been partially attributed to slamming.
Literature Review
The history of research into slamming and impact loads is relatively recent mainly because it is, as far as naval architecture is concerned, predominantly a high speed phenomenon. The general problem of hydrodynamic impact has also been studied extensively motivated in part by its importance in ordnance and missile technology. A large number of mathematical models have been developed for cases of simple geometry such as spheres and wedges. These models have been well supported by experiment. Unfortunately, the special case of wave impact has not been studied extensively.
The early impact theory of von Karman (1929) was based on the principle of conservation of momentum throughout the impact. Prior to impact all momentum is associated with the body, but as the wedge becomes immersed part of the momentum is imparted to a mass of water which is in contact of the bottom and which has the same velocity as the bottom. With a constant momentum, increased mass required a decreased velocity for the system and the change of momentum of the body alone defined the force on the body. von Karman proposed an attached or virtual mass equal to the mass of a half-cylinder of water having a diameter equal to the instantaneous width of the wedge in the plane of the undisturbed water surface. While von Karman’s paper was the first, mathematical details came with Wagner’s (1931) work published three years later. The basic idea of the mathematical treatment proposed by Wagner can still be recognized in recent papers. In his somewhat difficult-to-read publication he solved the impact problem of the two-dimensional symmetric wedge of small deadrise angle, discussed the concept of similarity solution, computed the piled up water surface, the pressure distribution, spray thickness, gave the equation of a constant force bottom and introduced a deadrise angle correction factor. It can be stated without exaggeration that von Karman’s physical picture and Wagner’s mathematical treatment are still valid and useful, and form the basis of most later papers.
When considering the various forces acting on offshore drilling platforms at sea, an important class of forces for which there is no extensive history of study are the impact forces that act on horizontal structural members in the so called splash zone. The splash zone is a region wherein the particular horizontal members of the platform are not usually considered to have continuous contact with the waves, but which are located at a height relative to the mean water surface so that only occasional contact with the water will occur (see Figure 1). The nature of the forces that occur during such contacts is essentially impulsive, and thus somewhat similar to the forces associated with the often-encountered slamming of ships, where the relative emersion and immersion of a portion of the ship bow region with respect to the sea surface produces impacts on the ship.
Wave forces on structures composed of slender members are traditionally calculated on the basis of the classical Morison equation, in which the force at any section of a member is expressed directly in terms of the fluids kinematics that would occur at that section’s location (Morison et al., 1950). However, the Morison equation is generally applicable only to portions of an immersed body that are in full contact with the water continually, and as such there is a question as to whether that particular approach can be considered applicable to the present impact force problem for horizontal members.
Kaplan and Silbert (1976) developed a mathematical model for determining time histories of vertical impact forces on platform horizontal structural members in the splash zone.
Dalton and Nash (1976) conducted slamming experiments with a 1.27 cm diameter cylinder with small amplitude waves generated in a laboratory tank. But their data exhibited large scatter and showed no particular correlation with either the predictions of hydrodynamic theory or identifiable wave parameters.
Miller (1977) developed a computer model for the vertical wave force on an instrumented cylinder which behaves as a two degree-of-freedom dynamic system. The slamming force was assumed to increase linearly to a peak value over a specific rise time, and to decrease linearly to zero over a specific decay-time. The drag and inertia components were assumed to act only when the cylinder was fully immersed and were computed from constant drag and inertia coefficients. The resulting combined force exhibits discontinuities in magnitude and the buoyancy component had to be reduced when the water surface was reducing in order to avoid an unrealistic dynamic response during that stage. Miller also identified loading regimes associated with wave slamming and described slamming tests on a cylinder in waves. Tests conducted for 3 cylinder elevations indicated an average value of CS0 of 3.6, although there was appreciable scatter in the results. He concluded that this was consistent with the theoretical value of π and attributed the observed higher value to dynamic amplification effects. The effects of the slamming force rise time on the dynamic response were illustrated by simulating numerically the vertical wave force using a dynamic analogue and comparing the computed force traces with the experimental records.
Faltinsen et al. (1977) investigated the load acting on horizontal circular cylinders (with end plates and length-to-diameter ratios of about 1) which were forced with constant velocity through an initially calm free surface. They found that the slamming coefficient ranged. They also conducted experiments with flexible horizontal cylinders and found that the analytically predicted values were always lower (50 to 90 per cent) than those found experimentally.
Sarpkaya (1978) conducted slamming experiments with harmonically oscillating flow impacting a horizontal cylinder and found that: (a) the dynamic response of the system is as important as the impact force (that is, one cannot be determined without accounting for the other); (b) the initial value of the slamming coefficient is essentially equal to its theoretical value of ; (c) the system response may be amplified or attenuated, depending on its dynamic characteristics; (d) the buoyancy-corrected normalized force in the drag-dominated region reaches a maximum at a relative fluid displacement of about 1.75; and (e) roughness increases the rise time of the force and tends to decrease the amplification factor.
Kaplan (1979) described some of the problems associated with the measurement of high frequency impact forces on offshore structures. The effects of various elements of the measuring and data processing systems on the data, as well as the correlation with theory, were presented in detail. Theoretical representations of the various physical mechanisms contributing to the total forces, both vertical and horizontal, were described and exhibited in the results. Comparisons between theory and experiment were presented in the form of time history characteristics as well as analysis of peak values for use in design estimation. He found that the slamming coefficient ranged from 1.88 to 5.11, with just as many values above the theoretical value of π as below, and a mean value of CS = 2.98.
Miller (1980) has shown that for most structures the current practice of applying a constant slamming coefficient of 3.5 is conservative when estimating extreme stresses. For fatigue estimation this appears to be the case irrespective of the member geometry.
Campbell and Weynberg (1980) measured spanwise and circumferential pressures in addition to the vertical force on horizontal and inclined cylinder driven through a still water surface. They observed that the slamming force was predominant for tests involving a Froude number (Fr = U/ , where U is the fluid velocity, g is the gravitational acceleration and D is the cylinder diameter) higher than 0.6. They also indicate that the slamming force was masked by the dynamic response of the force transducer and the scatter in the observed data was the result of variable rise-times which were sensitive to small variation in the slope of the cylinder. It was also noted that drips from the cylinder had a significant effect on the response. They summarized their results by proposing an empirical equation that relates the slamming coefficient and cylinder submergence, and which uses CS0 = 5.15. This equation is independent of the Froude number and was not corrected for buoyancy effects.
Cointe and Armand (1987) used the method of matched asymptotic expansions to solve the boundary value problem for water-cylinder impact. They too conclude that CS0 is 2π rather than π as given by some of the earlier theories.
Greenhow and Li (1987) reviewed a number of different formulations for evaluating the added mass of a horizontal circular cylinder moving near the free surface and conclude that the effects of free surface deformation on the slamming coefficient is significant and must be included in any theoretical treatment. They recommend two different methods to calculate the added mass for small and large cylinder submergence respectively which both indicate a CS0 value of 4π/3.
Isaacson and Subbiah (1990) described random wave forces acting on a fixed, slender, horizontal circular cylinder in the vicinity of the free surface, taking account of the intermittency of submergence and wave slamming. They derived expressions for the probability density and the mean of the force maxima, and calculated the expected value of the single largest force maximum occurring within a given duration. These predictions were based on the assumptions of linear wave theory and a narrow-band wave spectrum. They presented useful numerical results and discussed the effects of intermittent submergence and wave slamming.
Miao (1990) computed the bending stress on a flexible cylinder subjected to water impact. The hydrodynamic force was computed as the sum of the momentum, drag and buoyancy forces and the equation of motion was solved for various end fixity conditions using the mode superposition approach. Computed bending stresses compared well with his experimental observation of slamming on a cylinder driven into still water. Miao also reported results from experiments on a 1.52 m long flexible horizontal cylinder which was driven at a constant velocity through a stationary water surface. He proposed an expression for the variation of CS with submergence which indicates a CS0 value of 6.1 and also concluded that for typical truss members in heavy seas, thy dynamic application of the response and the induced stresses ranged from 0.3 to 0.6 due to the short impulse times observed in the experiments.
Chan et al. (1991) presented the results of an experimental study of plunging wave action on a large horizontal cylinder in the splash zone. Impact pressures were found to range from localized impulsive pressures with time scales in the range of 0.001T to synchronous low frequency pressure oscillations with oscillation time scale around 0.01T (T being the characteristic wave period). The peak impact force was about c2R per unit length of the cylinder (where ρ is the water density, C is the characteristic phase speed of the wave and R is the radius of the cylinder. Overall, the incident wave profile trajectory and the entrapped air were found to influence the impact load significantly.
Isaacson and Prasad (1992 and1994) described a numerical and experimental study of the vertical force acting on a section of a fixed, horizontal circular cylinder located near the free surface. The two-dimensional case of unidirectional waves propagating in a direction orthogonal to the cylinder axis has been considered. Numerical models are developed for the time-varying vertical force on fixed and dynamically responding cylinders on the basis of specified empirical force coefficients. As an alternative to the conventional approach involving the slamming coefficient, the suitability of an impulse coefficient which combines the impact force and rise time into a single dimensionless quantity has also been examined.
Khalil and Ali (1994) conducted an investigation into wave slamming loads on horizontal circular cylinders. They provided an analytical approach for deriving an expression for the slamming coefficient. Subsequently, they developed a computer code based on the theoretical analysis and generated computational results. The results of computation were plotted graphically with a view to explaining the salient features of wave slamming on cylindrical structures like the braces between legs of an offshore platform.
Cortel and Grilli (2006) studied the impact on cylindrical piles of extreme waves, generated by directional wave focusing. Waves were numerically modeled based on a boundary element discretization of fully non-linear potential flow equations with free surface elevation. Finally, the full loading on a cylindrical tower structure, due to a freak wave, was determined.
Zylinsky (2009) performed finite element analysis of wave slamming on offshore structure. He focuses on the analysis of wave slamming on floating platform column. Dynamic analysis has been performed with non-linear finite element method software package ABAQUS.
Khalil, Tarafder and Akter (2009) presented a mathematical model for the wave impact forces acting on a horizontal circular cylinder from the instant of impact to full immersion. They derived an expression for the slamming coefficient as a function of the Keulegan-Carpenter number, wave amplitude, diameter of the circular cylinder, instantaneous height of the wave surface above the mean water level, depth of immersion and added mass per unit length of the cylinder. A computer code is developed on the basis of the aforesaid theoretical analysis. The computational results are plotted in order to show the variation of the slamming coefficient with the Keulegan-Carpenter number, non-dimensional immersed area, non-dimensional added mass and relative submergence.
Present Research
The purpose of the present research is to analyse the wave impact forces acting on a fixed, slender, horizontal circular cylinder located above the still water level. It presents a new mathematical model for the impact forces acting on a horizontal circular cylinder from the instant of impact to full immersion. Two different expressions are developed for the slamming coefficient. The first expression is a function of the Froude number whereas the second expression is a function of the Keulegan-Carpenter number. It may be noted that the Froude number and the Keulegan-Carpenter number are two important dimensionless parameters associated with wave mechanics. These two parameters are used extensively in the design of offshore structures. Thus the expressions of the slamming coefficient which include the aforesaid dimensionless parameters are expected to be very useful in the analysis of wave impact forces on offshore structures.
A computer program is developed on the basis of the aforesaid theoretical analysis. The computational results are plotted in order to show the variation of the slamming coefficient with the Froude number, Keulegan-Carpenter number, cylinder diameter-to-wave amplitude ratio, non-dimensional immersed area, non-dimensional added mass, relative submergence and wave steepness. In order to check the validity of the mathematical model developed, the present computational results are compared with the theoretical and experimental results of other investigators.
THEORETICAL FORMULATION
The general case of hydrodynamic impact is usually described by using incompressible potential flow theory. The compressibility of water and air and the cushioning effect of air (air boundary layer, depression of the water surface just before impact, etc.) are ignored. For a moving body with mass , and velocity , impacting a quiescent surface, the system momentum is . Neglecting non-conservative forces, the momentum of the system is unchanged during penetration. However, the mass of the system increases because of the fluid set in motion near the body. Also known as added mass, results in reducing the velocity. Thus, the system momentum after penetration is . The impact force at any instant is a function of the added mass and its time derivative . Therefore, the solution requires knowledge of the added mass and its time derivative. The determination of the added mass is not a simple matter and the results depend on the assumptions made (Moran, 1965). The following analysis is based on the added mass calculated by Taylor (1930).
This section presents a two-dimensional analysis of the hydrodynamic forces acting on a fixed horizontal circular cylinder in the vicinity of the free surface, taking account of the intermittent submergence and wave slamming. The following assumptions are made in the present theoretical analysis:
- The wave system propagates in a direction normal to the axis of the horizontal circular cylinder.
- The motion of the free surface is assumed to be a sinusoidally oscillating one.
- The water is assumed to be inviscid, incompressible and the flow is irrotational.
- The diameter of the cylinder is assumed to be much smaller than the wave length.
- The free surface on the small circular cylinder is assumed to be flat.
Let us now analyse the hydrodynamic forces acting on a fixed horizontal circular cylinder located near the free surface, taking account of the intermittent submergence and wave slamming. It is assumed that the wave system propagates in a direction normal to the horizontal cylinder. The particular problem considered here is shown in Figure 2, where h is the height of the bottom of the cylinder above the mean water level, is the instantaneous height of the wave surface above the mean water level, and S is the instantaneous depth of cylinder immersion and is the immersed area.
The total vertical force acting on the immersed cylinder section is made up of a buoyant force and a hydrodynamic force of inertial nature due to the body-wave interaction. The force on the circular cylinder can be expressed as where is the force of buoyancy and is the inertial hydrodynamic force.
The tendency of a fluid to uplift a submerged body, because of the upward thrust of the fluid, is known as the force of buoyancy or simply buoyancy. It is always equal to the weight of the fluid displaced by the body. Thus the force of buoyancy is given by where represents the density of water, g is the gravitational acceleration and is the immersed area of the cylinder.
The inertial hydrodynamic force is obtained by generalizing the relations given by Kaplan (1957) and Kaplan and Hu (1959) for body-wave interaction, which leads to the expression in which represents the vertical added mass per unit length of the circular cylinder. The first and second derivatives of with respect to time are denoted by and which represent the vertical wave velocity and vertical wave acceleration respectively. The first term in Equation arises due to the spatial variation of the wave characteristics and the second term arises from the time rate of change of fluid momentum associated with the immersed portion of the cylinder section.
The expression for can be simplified as:
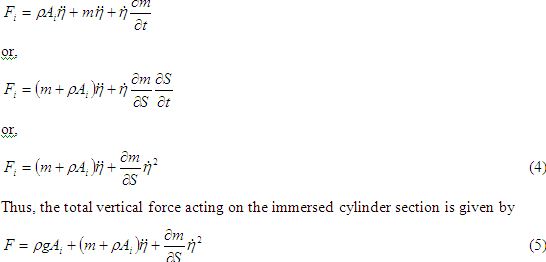
This expression holds good only if there is water contact and resulting immersion of a portion of the circular section. An evaluation of this force expression, which is a two-dimensional force per unit length of the cylinder, requires determination of the immersed area and the vertical added mass , as well as its rate of change with immersion .
Referring to Figure 2, we can perform a simple geometrical analysis to obtain an expression for the immersed area of the horizontal circular cylinder. Let O be the centre and D be the diameter of the circular cylinder. AB is the water level and OC is drawn vertically downwards from the centre. ACB is the immersed area of the circular cylinder and a is half of the angle subtended at the centre by the water level.
From the geometry of the figure, we find that
OA = OB =R
where R is the radius of the circular cylinder.
Table 1. Values of the slamming coefficient obtained by different researchers
| Investigators (year) | Nature of investigation | Value of the slamming |
coefficient Fabula (1957)TheoreticalCS0 = 6.28Dalton and Nash (1976)ExperimentalCS0 = 1.0 – 6.4Kaplan and Silbert (1976)TheoreticalCS0 = 3.14Holmes et al. (1976)ExperimentalCS0 = 0.4 – 2.9Faltinsen et al. (1977)Theoretical
ExperimentalCS0 = 3.14
CS0 = 4.1 – 6.4Miller (1977)ExperimentalCS0 = 1.0 – 6.4Srpkaya (1978)Theoretical
ExperimentalCS0 = 3.14
CS0 = 1.57 – 5.34Arhan et al. (1978)ExperimentalCS0 = 2.4 – 6.9Campbell and Weynberg (1980)ExperimentalCS0 = 1.0 – 6.4Armand and Cointe (1986)TheoreticalCS0 = 6.28Miao (1988)ExperimentalCS0 = 6.1Isaacson and Prasad (1992)ExperimentalCS0 = 1.0 – 6.4Present study (2011)TheoreticalCS0 = 3.142
It is observed that the value of the slamming coefficient at the instant of impact (CS0) obtained from the present theoretical model is equal to 3.142 which is exactly the same as those found by Kaplan and Silbert (1976), Faltinsen et al. (1977) and Sarpkaya (1978). However, experimental studies have yielded values of CS0 which exhibits a considerable degree of scatter, ranging from about 1.0 to 6.4 (Dalton and Nash, 1976; Miller, 1977; Sarpkaya, 1978; Campbell and Weynberg, 1980 and Isaacson and Prashad, 1992). Thus the present theoretical model yields a value of the initial slamming coefficient which agrees well with the theoretical and experimental results of previous researchers.
CONCLUSIONS
This thesis presents a two-dimensional analysis of the hydrodynamic forces acting on a fixed, horizontal circular cylinder in the vicinity of the free surface, taking account of the intermittent submergence and wave slamming. The theoretical analysis and computational results lead to several important conclusions which may be summarized as follows:
(a) Two new expressions are derived for the slamming coefficient, the first expression as a function of the Froude number and the second expression as a function of the Keulegan-Carpenter number. The Froude number and the Keulegan-Carpenter number are two important dimensionless parameters associated with wave mechanics. These two parameters are used extensively in the design of offshore structures. Thus the expressions of the slamming coefficient which include the aforesaid dimensionless parameters are expected to be very useful in the analysis of wave impact forces on offshore structures.
(b) The non-dimensional added mass of the circular cylinder is observed to increase steadily with relative submergence (S/D).
(c) The rate of change of added mass f3(θ) of the circular cylinder attains a value of 3.142 when relative submergence (S/D) is equal to zero. However, f3(θ) decreases rapidly as the relative submergence increases and attains the minimum value when S/D = 0.75, but then it increases again with any further increase of relative submergence.
(d) The slamming coefficient can be expressed through the diameter of the circular cylinder, wave amplitude, depth of immersion of the cylinder, instantaneous height of the surface wave above the mean water level and the added mass per unit length of the cylinder.
(e) At the instant of impact, the slamming coefficient (CS0) is equal to 3.142 for all Froude numbers and Keulegan-Carpenter numbers. But as the relative submergence of the cylinder increases, the value of the slamming coefficient sharply decreases at the beginning and then increases again. The sudden decline of the slamming coefficient after the initial impact indicates the impulsive nature of the slamming force. The slamming coefficient attains a maximum value when the Froude number or Keulegan-Carpenter number are minimum and then it decreases continuously as these two numbers increase.
(f) The slamming coefficient decreases as the height of the circular cylinder from the mean water level increases. Physically, it means that a horizontal circular cylinder experiences a greater wave impact force if it is located at a relatively lower position from the mean water level.
(g) It is necessary to mention that the proposed theoretical model has been developed without taking viscosity into account. But water is a viscous fluid. This is why some differences are observed between the present theoretical results and previously published experimental data. Evidently, the predictions of the present theoretical model are not valid beyond very small values of relative submergence.
(h) The slamming coefficient, and consequently the impact force, is of an impulsive nature beginning with a finite value (equal to 3.142) at the instant of impact. Since viscous forces are neglected in the analysis of impact forces, one would expect the solution to deviate from the actual situation as the cylinder becomes more deeply immersed. Where this becomes the case the slamming coefficient can only be determined experimentally.
(i) The present theoretical model predicts the slamming coefficient for a horizontal circular cylinder from the instant of impact to full immersion. In order to check the validity of the present theoretical model, the computational results are compared with the theoretical and experimental results of other investigators and satisfactory agreement is found for small values of relative submergence.