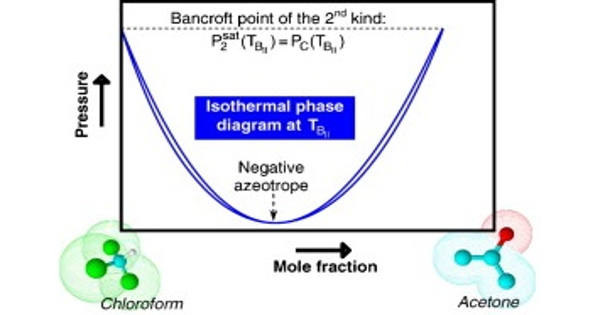
In a binary system, a Bancroft point is the temperature at which an azeotrope occurs. Although vapor-liquid azeotropy is impossible for binary systems that are rigorously described by Raoult’s law, azeotropy is unavoidable for real systems at temperatures where the components’ saturation vapor pressures are equal. A temperature of this type is known as a Bancroft point. However, such a point does not exist in all azeotropic binary systems. A Bancroft point must also be located within the valid temperature ranges of the Antoine equation.
A Bancroft point is the point at which the vaporization curves of two components in a (P, T) plane intersect. There are numerous binary systems for which such a point exists. The binary system methanol+acetone is one example.
The Bancroft point is the point at which two substances’ saturation vapor pressure curves intersect. Another component has a higher vapor pressure above the point than below the point. There is also talk of a vapor pressure inversion. The Bancroft point occurs only when the saturation vapor pressures of the components are of a similar order of magnitude. The Bancroft point is significant because it clearly indicates the azeotropic behavior of a binary mixture of the two substances.
A Bancroft point (a term that is rarely used in my circles) is the temperature at which the liquid and vapor phases of a binary system have the same composition. There are no counter examples because this is the definition of an azeotrope. This may not be true if you have a different definition of a Bancroft point.
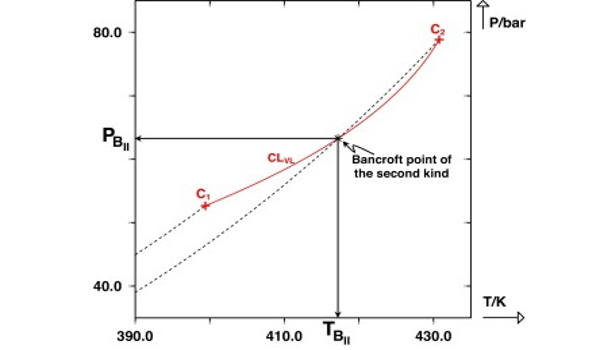
The possible shapes of continuous critical loci for binary systems with a negative azeotrope that persists up to the critical region are reviewed and discussed extensively. The existence of an intersection point – in a (P, T) projection – between the critical line and one of the vaporization curves was shown to be a sufficient condition for a binary system to exhibit a critical azeotrope. Bancroft’s rule, which previously stated that the existence of an intersection point between two vaporization curves in a (P, T) plane was a sufficient condition for observing azeotropic behavior, was thus upgraded to include the crossing of the critical line and one of the vaporization curves. A point of this type was logically referred to as a Bancroft point of the second kind.
Bancroft point is named after Wilder Dwight Bancroft.
Information Source: