A random variable (stochastic variable) is a variable with an undefined value or a function that assigns values to each of the results of an experiment. Since a random variable may take on various values, a letter (e.g. variable ‘X’) is usually called. Depending on the case, the random variable takes on various values. Each estimation of the random variable has a likelihood or rate related with it. Arbitrary factors are frequently utilized in econometric or relapse investigation to decide measurable connections among each other.

The possible values of a random variable may reflect the possible results of an experiment yet to be conducted, or the possible results of a previous experiment whose already existing value is uncertain (for example, due to imprecise measurements or quantum uncertainty). Random variables are used in probability and statistics to measure the effects of a random event, and can thus take on several values. They may likewise adroitly speak to either the consequences of an “impartially” random cycle, (for example, rolling a bite the dust) or the “emotional” arbitrariness that outcome from deficient information on an amount.
To be measurable, random variables are needed and are usually real numbers. For instance, after three dices are rolled, the letter X may be assigned to represent the sum of the resulting numbers. In this scenario, X maybe 3 (1 + 1 + 1), 18 (6 + 6 + 6), or anywhere between 3 and 18, because the maximum number of deaths is 6 and the lowest number is 1. A random variable that is discrete is a variable that represents numbers identified by counting. For instance: the number of marbles in a container, the number of understudies present, or the number of heads while flipping two coins.
Random variables are categorized into variables that are discrete and continuous. The type of potential values that can be taken by each variable is the key difference between the two groups. An undefined value that can be determined is the variable in an algebraic equation. The equation 10 + x = 13 illustrates that the real value of x, which is 3, can be determined. In addition, the form of a (random) variable implies a specific method of finding a function for the distribution of probability.
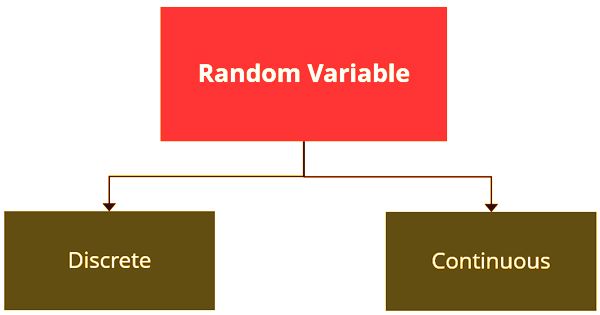
Two Types of Random Variable
The importance of the probabilities appointed to the possible estimations of an arbitrary variable isn’t essential for the likelihood hypothesis itself, yet is rather identified with philosophical contentions over the translation of likelihood. Random variables can be allocated to assets in the corporate world, such as the average price of an asset for a defined period of time, the return on investment over a stated number of years, the expected turnover rate for a corporation for the next six months, etc.
Discrete: A discrete random variable is a (random) variable whose qualities take just a limited number of qualities. Consider an analysis where a coin is thrown multiple times. In the event that X speaks to the occasions that the coin comes up heads, at that point X is a discrete random variable that can just have the qualities 0, 1, 2, 3 (from no heads in three progressive coin throws to all heads). No other worth is feasible for X.
Continuous: Within a given range or interval, continuous random variables can represent any value and can take on an infinite number of possible values. The returns of stocks are one such example of a continuous variable. An infinite number of potential values (as percentages) can be used for the returns. For the above cause, the likelihood of a certain result for the continuous random variable is zero.
In any case, there is consistently a non-negative likelihood that a specific result will exist in the span between two qualities. The area of an arbitrary variable is known as an example space. It is deciphered as the arrangement of potential results of an irregular wonder. If they want to measure the likelihood of an adverse occurrence happening, risk analysts assign random variables to risk models. These factors are described using methods such as scenario and sensitivity analysis tables that are used by risk managers to make risk reduction decisions.
A blended random variable is an arbitrary variable whose total dispersion work is neither piecewise-consistent (a discrete arbitrary variable) nor wherever nonstop. In money, irregular factors are generally utilized in monetary displaying, situation investigation, and danger of the executives. The probabilities of the variables in financial models and simulations reflect the probabilities of random phenomena that affect the price of a security or determine the level of risk of an investment. The result of a coin flip is a common example of a random variable. Consider a distribution of probabilities in which it is not equally probable that the consequences of a random event will happen. In the event that arbitrary variable, Y, is the quantity of heads we get from flipping two coins, at that point Y could be 0, 1, or 2. This implies we could have no heads, one head, or the two heads on a two-coin throw.
Information Sources: