“Variation” defines a concept that deals with variability in mathematics. Variation is defined by any change in some quantity due to change in another. We often come across with different types of variation problems in mathematics. Variation problems involve fairly simple relationships or formulas, involving one variable being equal to one term. Here follow the most common kinds of variation.
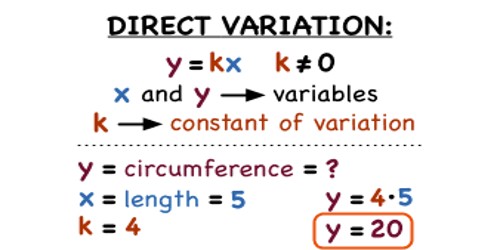
The constant of variation in a direct variation is the constant (unchanged) ratio of two variable quantities. In the following equation, y varies directly with x, and k is called the constant of variation: y = kx
Direct Variation
When two variables change in proportion it is called as, direct variation. In direct variation, one variable is constant times of other. If one variable increases other will increase, if one decrease other will also decease. This means that the variables change in the same ratio which is called a constant of variation.
Direct variation is the simplest type of variation and in practical life, we can find many situations which can be co-related with direct variation.
If two variables A and B are so related that when A increases ( or decreases ) in a given ratio, B also increases ( or, decreases ) in the same ratio, then A is said to vary directly as B ( or, A is said to vary as B ).
This is symbolically written as A ∝ B (read as ‘A varies as B’ ).
Suppose a train moving at a uniform speed travels d km. in t minutes. Now, consider the following table:
d (km) 24 12 48 36
t (min) 30 15 60 45
Like in a math examination if for one problem solving we can score 10 numbers, so five problems solving we can get 50 numbers. This can be explained by a direct variation equation. If T denotes total numbers scored, N denotes numbers of the problem solved and K denotes numbers can be scored for solving a problem, then the direct variation equation for this situation will be T = KN.
As the numbers for a problem can be scored is fixed, it is a constant = K = T/N = 10
For solving 5 problems total numbers scored T = KN = 10 x 5 = 50.
From the above example, we can understand that the ratio of two variables is a constant K and T, N are the variables which changes in proportion to a value of constant.
Direct variation can be by a linear equation Y = KX where K is a constant. When the value of constant is higher, the change of variable Y is significantly for a small change of X. But when the value of K is very small, Y changes very less with a change of X. For this case, K is equivalent to the ratio of change of two variables. So (σY/σX) = K when K is very small.
Now we will solve some problems on direct variation:
- If P varies directly as Q and the value of P is 60 and Q is 40, what is the equation that describes this direct variation of P and Q?
Solution:
As P varies directly with Q, ratio of P and Q is constant for any value of P and Q.
So constant K = P/Q = (60/40) = 3/2
So the equation that describes the direct variation of P and Q is P = 3/2 Q.
- If a car runs at a constant speed and takes 3 hrs to run a distance of 180 km, what time it will take to run 100 km?
Solution:
If T is the time taken to cover the distance and S is the distance and V is the speed of the car, the direct variation equation is S= VT where V is constant.
For the case given in the problem,
180 = V × 3 or V = 180/3 = 60
So the speed of the car is 60kmph and it is constant.
For 100 km distance
S = VT or 100 = 60 × T
T = 100/60 = 5/3 hrs = 1 hr 40 mins.
So it will take 1 hr 40 mins time.
Information Source: