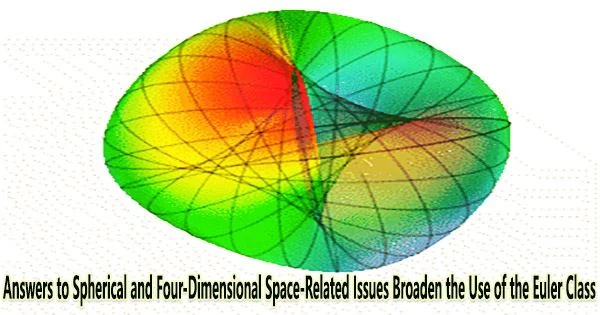
In cooperation with Purdue University, mathematicians from EPFL have answered a 30-year-old query about spheres and four-dimensional spaces. The “Euler class,” one of the most effective tools for comprehending complex spaces, is given new insight by the results.
Spheres are three-dimensional objects that are completely round and have no edges or corners. They can be described by their radius, which is the distance from the center of the sphere to any point on its surface.
A four-dimensional space, on the other hand, is a mathematical concept that extends the familiar three-dimensional space we live in to include an additional dimension. This fourth dimension is typically referred to as “time,” and is used to describe the movement of objects in our world.
One of the most effective mathematical methods for breaking complex spaces down into simpler parts is the Euler class. It bears the name of Swiss mathematician Leonard Euler, who initially thought of the concept.
“Just like something as complex as DNA is ultimately made of simple atoms, it is how these simple pieces are assembled that contains the important information rather than the pieces themselves,” says Professor Nicolas Monod, who leads the Ergodic and Geometric Group Theory research unit at EPFL. His group joined forces with colleagues at Purdue University to solve an old question about spheres.
The answer has been published the mathematics journal Inventiones mathematicae.
A very curious thing happened when we set out to solve this problem. If it remained unsolved for so long, it is perhaps because none of the classical methods used to understand spaces seemed to be able to crack this specific question about 4 dimensions. Instead, we turned to an unlikely source for inspiration: tossing coins.
Professor Nicolas Monod
In 1958, Fields medalist John Milnor noticed a problem when trying to build spaces using only circles and two-dimensional surfaces: there was a limit on how complicated the Euler class can be in two dimensions.
Mathematicians soon discovered that Milnor’s “complexity bound” did not hold for spaces in all dimensions as the discovery swiftly grew into a whole field of study in higher dimensions.
Monod explains, “A question that had remained open for decades was, what about gluing spheres on 4-dimensional spaces? Is there also here a limit on how they fit together?”
He continues: “Gluing spheres on 4-dimensional spaces is a particularly important construction because this is precisely how the very first ‘exotic spheres’ have been constructed.”
This four-dimensional problem has proven impossible to answer using traditional methods of understanding spaces. The Bernoulli process, which bears the name of Swiss mathematician Jacob Bernoulli, served as an inspiration for the EPFL mathematicians. In order to arrive at the final solution, the Euler class, spheres, and the Bernoulli process a model of coin tossing were all used.
“A very curious thing happened when we set out to solve this problem,” says Monod. “If it remained unsolved for so long, it is perhaps because none of the classical methods used to understand spaces seemed to be able to crack this specific question about 4 dimensions. Instead, we turned to an unlikely source for inspiration: tossing coins.”
As a game with a 50-50 chance to guess the right side of a coin, this might seem very simple, but its simplicity is misleading.
“The Bernoulli process already includes many of the advanced features of probability theory when we set out to repeat the toss more and more often,” says Monod. “In fact, the Central Limit Theorem which is a kind of Law of Large Numbers even tells us that this simple model can emulate many of the most complicated random phenomena of nature if we are willing to toss enough coins for long enough.”
Although probability and randomness may not seem to have anything to do with the study of higher spatial dimensions, mathematics is both a creative art and a science.
“Earlier this year, we published the discovery that Bernoulli’s random coin games can help solve some difficult algebraic questions, very much non-random questions,” says Monod. “This has now been combined with the study of spheres and the Euler class to finally solve the old question about 4-dimensional spaces: no, there is no limit at all to the size of the Euler class for spheres in four dimensions.”
“So the coins came to the rescue of algebra and geometry, and Bernoulli visits the Euler class: indeed, mathematicians do things differently,” he concludes.
Understanding and visualizing four-dimensional spaces can be difficult for humans, as our brains are not accustomed to processing information in more than three dimensions. However, mathematical models and computer simulations can be used to study and explore the properties of four-dimensional spaces, and their applications in physics, engineering, and other fields.