Exponential growth is when information ascends throughout some stretch of time, making an upwards moving bend on a chart. It happens when the immediate pace of progress (that is, the subordinate) of an amount regarding time is corresponding to the actual amount. When a formula involves a power (or an exponent) in mathematics, the calculation increases exponentially. If hens lay eggs three times a year and the number of eggs triples per year, the second year will have 27, and the third year will have 81. Compounding produces exponential returns in finance; for example, savings accounts with a compounding interest rate will rise exponentially.
Build revenue is great for financial backers, as they can expand their total assets over the long run utilizing a limited quantity of income. Portrayed as a capacity, an amount going through remarkable development is an outstanding capacity of time, that is, the variable addressing time is the example (as opposed to different sorts of development, like quadratic development). Compounding is one of the most strong forces in the financial world. This idea enables investors to generate large amounts of money with a small initial investment. Exponential growth is often seen in savings accounts with a compound interest rate.
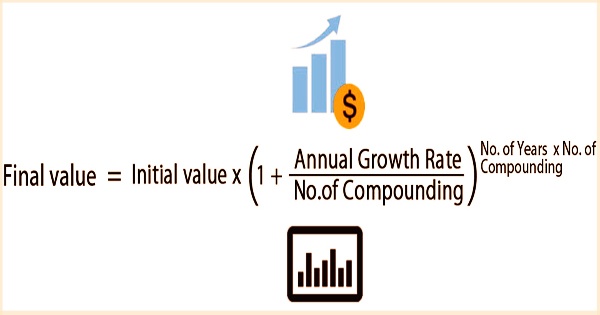
The formula for the exponential growth of a variable x at a given growth rate r over time t in discrete intervals (that is, at integer times 0, 1, 2, 3,…) is
xt = x0 (1 + r)t
where x0 is the value of x at time 0.
When a person invests money in high-yield savings accounts for a long time, the investor will earn compound returns due to exponential growth in the financial world. It is an illustration of how speculations can develop dramatically with a minimal introductory expense. In genuine cases, beginning outstanding development regularly doesn’t keep going forever, rather easing back down in the end because of furthest cutoff points brought about by outer factors and transforming into strategic development.
Exponential growth can be calculated using the following steps:
- Step 1: Determine the starting value for which the final value must be determined first. For example, in a time value of money calculation, it may be the present value of money.
- Step 2: Next, try to figure out the annual growth rate, which can be determined depending on the application form. For example, if the formula is used to measure a deposit’s future value formula, the growth rate will be the estimated rate of return based on the market situation.
- Step 3: Now it’s time to find out how long the growth will last in terms of years, i.e. how long the valuation will be on such a steep growth curve.
- Step 4: Now figure out how many compounding times there are in a year. Compounding may be done on a quarterly, half-yearly, annual, or continuous basis, among other options.
- Step 5: Finally, the final value is calculated by compounding the initial value (step 1) using an average growth rate (step 2), the number of years (step 3), and the number of compounding each year (step 4) as shown above.
Assuming the record gives a self multiplying dividends rate, the financial backer will get revenue on the head and premium installment got from the past period. While outstanding development is regularly utilized in monetary demonstrating, the fact of the matter is frequently more confounded. In the case of a savings account, exponential growth works well since the rate of interest is guaranteed and does not change with time. Compounding, in the context of investing, refers to the opportunity to increase one’s wealth exponentially over time by receiving interest on the extra profits earned from prior interest payments derived from the principal sum. It differs from simple interest in that it does not account for compounding.
In many ventures, this isn’t the situation. For example, securities exchange returns don’t easily follow long haul midpoints every year. Basic premium just pays revenue on the first head, excluding the income got over the lifetime of the monetary instrument. Since it is mainly used in the estimation of compound returns, it is important for a financial analyst to consider the principle of exponential growth equation. The monstrosity of the idea in money is exhibited by the influence of compounding to make an enormous aggregate with an altogether low beginning capital.
Exponential growth is frequently utilized in monetary displaying and albeit the idea is evident in a high-premium bank account, the justification that is on the grounds that loan costs watch out for not falter or change as much during various financial states. When it comes to stocks, though, the returns are not as consistent. Other methods of predicting long-term returns, such as Monte Carlo simulations, which use probability distributions to assess the likelihood of various possible outcomes, are becoming more common. Exponential growth models are more helpful to foresee speculation returns when the pace of development is consistent. Generally, dramatic development models are valuable in anticipating venture returns when the development rate is consistent and doesn’t waver much of the time.
Information Sources: