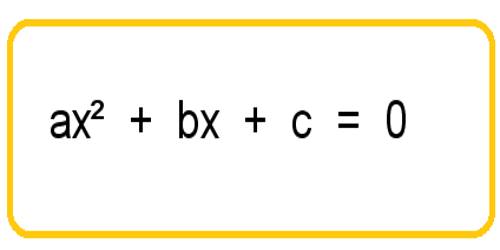Condition for Common Root of Quadratic Equations

Condition for Common Root of Quadratic Equations

A polynomial of the second degree is generally called a quadratic polynomial. In elementary algebra, the quadratic formula is the solution of the quadratic equation. There are other ways to solve the quadratic equation instead of using the quadratic formula, such as factoring, completing the square, or graphing. Using the quadratic formula is often the most convenient way.

If f(x) is a quadratic polynomial, then f(x) = 0 is called a quadratic equation.

An equation in one unknown quantity in the form ax² + bx + c = 0 is called quadratic equation.

Condition for one common root:

Let the two quadratic equations are a₁x² + b₁x + c₁ = 0 and a₂x² + b₂x + c₂ = 0

Now we are going to find the condition that the above quadratic equations may have a common root.

Let α be the common root of the equations a₁x² + b₁x + c₁ = 0 and a₂x² + b₂x + c₂ = 0. Then,

a₁α² + b₁α + c₁ = 0

a²α² + b₂α + c₂ = 0

Now, solving the equations a₁α² + b₁α + c₁ = 0, a²α² + b₂α + c₂ = 0 by cross-multiplication, we get

α²/b₁c₂ – b₂c₁ = α/c₁a₂ – c₂a₁ = 1/a₁b₂ – a₂b₁

⇒ α = b₁c₂ – b₂c₁/c₁a₂ – c₂a₁, (From first two)

Or, α = c₁a₂ – c₂a₁/a₁b₂ – a₂b₁, (From 2nd and 3rd)

⇒ b₁c₂ – b₂c₁/c₁a₂ – c₂a₁ = c₁a₂ – c₂a₁/a₁b₂ – a₂b₁

⇒ (c₁a₂ – c₂a₁)² = (b₁c₂ – b₂c₁)(a₁b₂ – a₂b₁), which is the required condition for one root to be common of two quadratic equations.

The common root is given by α = c₁a₂ – c₂a₁/a₁b₂ – a₂b₁ or, α = b₁c₂ – b₂c₁/c₁a₂ – c₂a₁

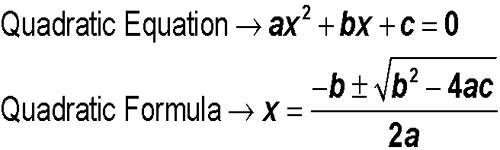
Note:

(i) We can find the common root by making the same coefficient of x² of the given equations and then be subtracting the two equations.

(ii) We can find the other root or roots by using the relations between roots and coefficients of the given equations

Condition for both roots common:

Let α, β be the common roots of the quadratic equations a₁x² + b₁x + c₁ = 0 and a₂x² + b₂x + c₂ = 0. Then

α + β = -b1/a1, αβ = c₁/a₁ and α + β = -b₂/a₂, αβ = c₂/a₂

Therefore, -b/a₁ = – b₂/a₂ and c₁/a₁ = c₂/a₂

⇒ a₁/a₂ = b₁/b₂ and a₁/a₂ = c₁/c₂

⇒ a₁/a₂ = b₁/b₂ = c₁/c₂

This is the required condition.

Information Source:

• www.math-only-math.com