The term “portfolio variance” actually refers to a statistical number used in modern investing theory to calculate the deviation of a portfolio’s average returns from its mean. It’s a risk indicator that shows how the aggregate actual returns of a portfolio’s assets fluctuate over time. It is a significant idea in the present-day venture hypothesis. To put it plainly, it decides the absolute danger of the portfolio. It very well may be determined dependent on a weighted normal of individual change and shared covariance.
The standard deviations of each asset in the portfolio, as well as the correlations of each security pair in the portfolio, are used to compute this portfolio variance statistic. The computation of portfolio variance thinks about the peril of individual resources as well as the connection between each pair of resources in the portfolio. A reduced portfolio variance is the outcome of a decreased correlation between stocks in a portfolio. The portfolio variance formula for two assets is represented mathematically as,
Portfolio Variance Formula = w12 × ơ12 + w22 × ơ22 + 2 × ρ1,2 × w1 × w2 × ơ1 × ơ2
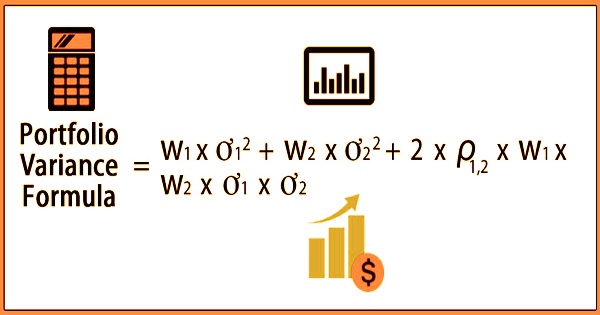
where,
wi = Portfolio weight of asset i
ơi2 = Individual variance of asset i
ρi,j = Correlation between asset i and asset j
In modern portfolio theory (MPT), the risk-axis of the efficient frontier is defined by portfolio variance (and standard deviation). As a result, statistical variance examines how assets in a portfolio tend to move in tandem. The overall standard of portfolio expansion is the determination of resources with a low or negative connection between’s one another. Portfolio fluctuation takes a gander at the covariance or connection coefficients for the protections in a portfolio.
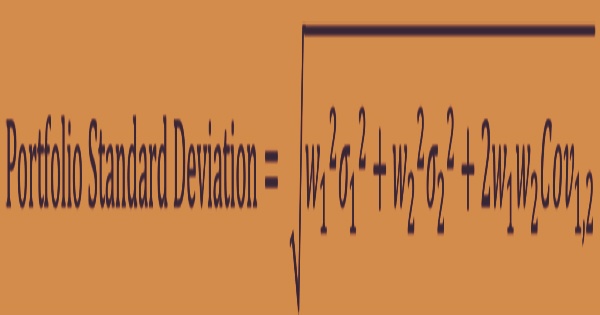
In general, a portfolio with a lower correlation between securities has a smaller variance. The square root of the portfolio variance can be used to compute the standard deviation of the portfolio variance:
Portfolio variance is determined by increasing the squared load of every security by its relating fluctuation and adding double the weighted normal weight duplicated by the covariance of all individual security sets. The following procedures can be used to calculate the portfolio variance formula for a certain portfolio:
- Step 1: To begin, compute the asset weight in the overall portfolio, which is obtained by dividing the asset value by the portfolio’s entire value. Wi denotes the weight of the ith asset.
- Step 2: Next, calculate each asset’s standard deviation, which is calculated using the mean and actual return of each asset. The ith asset’s standard deviation is denoted by i. Variance is defined as the square of the standard deviation, ơi2.
- Step 3: Determine the asset correlation, which simply represents the movement of one asset in respect to another asset. The correlation is denoted by ρ.
- Step 4: Finally, a weighted average of individual variance and mutual covariance is used to calculate the portfolio variance formula for two assets.
The formula for portfolio variance in a two-asset portfolio is as follows:
Portfolio variance = w12σ12 + w22σ22 + 2w1w2Cov1,2
Where:
w1 = the portfolio weight of the first asset
w2 = the portfolio weight of the second asset
σ1= the standard deviation of the first asset
σ2 = the standard deviation of the second asset
Cov1,2 = the covariance of the two assets, which can thus be expressed as p(1,2)σ1σ2, where p(1,2) is the correlation coefficient between the two assets
The portfolio variance formula is particularly crucial to understand because it is used in Modern Portfolio Theory, which is based on the idea that typical investors want to maximize their returns while minimizing risk, such as variance. As the quantity of resources in the portfolio develops, the terms in the equation for change increment dramatically. For instance, a three-resource portfolio has six terms in the difference computation, while a five-resource portfolio has 15.
Modern portfolio theory (MPT) is a framework for putting together a portfolio of investments. MPT is based on the assumption that rational investors desire to maximize returns while minimizing risk, which is sometimes assessed in terms of volatility. A financial backer for the most part seeks after what is called a proficient boondocks, and it is the least degree of hazard or instability at which the financial backer can accomplish its objective return. Regularly, financial backers would put resources into uncorrelated resources for bring down the danger according to Modern Portfolio Theory.
The standard deviation, which is determined as the square root of the variance, is commonly used to determine the risk level of a portfolio. When the data points are far from the mean, the variance is predicted to be high, which leads to a higher overall degree of risk in the portfolio. Portfolio managers, financial advisors, and institutional investors consider standard deviation as a crucial risk indicator. Standard deviation is frequently included in performance reports by asset managers.
Information Sources: