About Nash Equilibrium
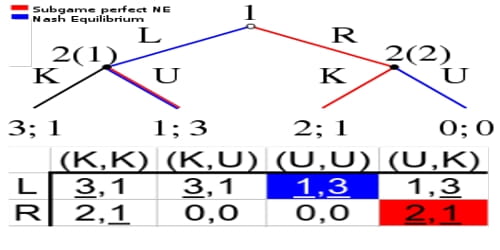
Nash Equilibrium is a concept of game theory where the optimal outcome of a game is one where no player has an incentive to deviate from his chosen strategy after considering an opponent’s choice. The concept is named after the American Mathematician and Nobel winning economist, John Nash, is a solution to a game involving two or more players who want the best outcome for themselves and must take the actions of others into account. Overall, an individual can receive no incremental benefit from changing actions, assuming other players remain constant in their strategies. A game may have multiple Nash Equilibrium or none at all. The reality of the Nash equilibrium of a game can be tested using experimental economics methods.
The Nash Equilibrium is the solution to a game in which two or more players have a strategy, and with each participant considering an opponent’s choice, he has no incentive, nothing to gain, by switching his strategy. In the Nash Equilibrium, each player’s strategy is optimal when considering the decisions of other players. Every player wins because everyone gets the outcome they desire. To quickly test if the Nash equilibrium exists, reveal each player’s strategy to the other players. If no one changes his strategy, then the Nash Equilibrium is proven.
Game theorists use the Nash equilibrium concept to analyze the outcome of the strategic interaction of several decision makers. In other words, it provides a way of predicting what will happen if several people or several institutions are making decisions at the same time and if the outcome depends on the decisions of the others. The simple insight underlying John Nash’s idea is that one cannot predict the result of the choices of multiple decision makers if one analyzes those decisions in isolation. Instead, one must ask what each player would do, taking into account the decision-making of the others.
The modern game-theoretic concept of Nash equilibrium is instead defined in terms of mixed strategies, where players choose a probability distribution over possible actions. The concept of the mixed-strategy Nash equilibrium was introduced by John von Neumann and Oskar Morgenstern in their 1944 book The Theory of Games and Economic Behavior.
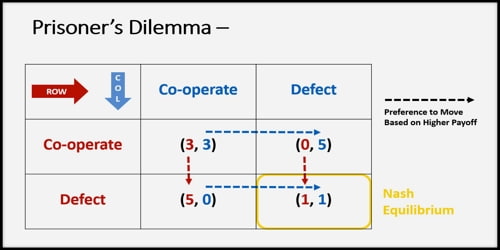
Prisoner’s Dilemma – The prisoner’s dilemma is a common situation analyzed in game theory that can employ the Nash Equilibrium. In this game, two criminals are arrested and each is held in solitary confinement with no means of communicating with the other. The prosecutors do not have the evidence to convict the pair, so they offer each prisoner the opportunity to either betray the other by testifying that the other committed the crime or cooperate by remaining silent. If both prisoners betray each other, each serves five years in prison. If A betrays B but B remains silent, prisoner A is set free and prisoner B serves 10 years in prison or vice versa. If each remains silent, then each serves just one year in prison. The Nash Equilibrium in this example is for both players to betray each other. Even though mutual cooperation leads to a better outcome if one prisoner chooses mutual cooperation and the other does not, one prisoner’s outcome is worse.
Informal Definition:
Informally, a strategy profile is Nash equilibrium if no player can do better by unilaterally changing his or her strategy. To see what this means, imagine that each player is told the strategies of the others. Suppose then that each player asks themselves: “Knowing the strategies of the other players, and treating the strategies of the other players as set in stone, can I benefit by changing my strategy?”
If any player could answer “Yes”, then that set of strategies is not Nash equilibrium. But if every player prefers not to switch (or is indifferent between switching and not) then the strategy profile is a Nash equilibrium. Thus, each strategy in Nash equilibrium is the best response to all other strategies in that equilibrium.
Formal Definition:
Formally, the Nash equilibrium is defined in terms of an n-player game where:
i = {1,…,n} players,
Si = the set of player strategies i ∈ I
gi = the set of goal functions S1 × … × Sn → ℝ.
Information Source: