Division of Negative and Positive Integers
Division of integers is the opposite operation of multiplying integers. It is the process by which one is trying to determine how many times a number is contained into another. Division of Integers is similar to division of whole numbers (both positive) except the sign of the quotient needs to be determined.
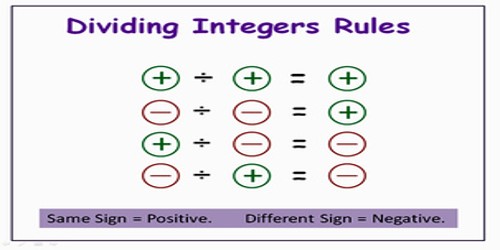
If both the dividend and divisor are positive, the quotient will be positive.
(+16) ÷ (+4) = +4
If both the dividend and divisor are negative, the quotient will be positive.
(-16) ÷ (-4) = +4
If only one of the dividend or divisor is negative, the quotient will be negative.
(+16) ÷ (-4) = -4 or (-16) ÷ (+4) = -4
In other words, if the signs are the same the quotient will be positive, if they are different, the quotient will be negative.
In division of integers we use the following rules:
Rule 1
The quotient of two integers either both positive or negative is a positive integer equal to the quotient of the corresponding absolute values of the integers.
(i) The quotient of two positive integers is positive. Here, we divide the numerical value of the dividend by the numerical value of the divisor.
For example; (+ 9) ÷ (+ 3) = + 3
(ii) The quotient of two negative integers is positive. Here, we divide the numerical value of the dividend by the numerical value of the divisor and assign (+) sign to the quotient obtained.
For example; (- 9) ÷ (- 3) = + 3
Thus, for dividing two integers with like signs, we divide their values and give plus sign to the quotient.
Rule 2
The quotient of a positive and a negative integer is a negative integer and its absolute value is equal to the quotient of the corresponding absolute values of the integers.
For example; (+ 16) ÷ (- 4) = – 4
Thus, for dividing integers with unlike signs, we divide their values and give minus sign to the quotient.
Information Source: