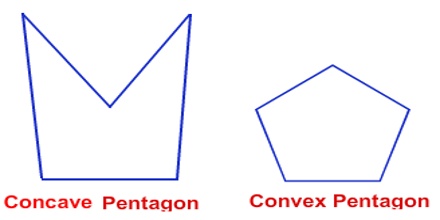
A pentagon is a 5-sided Polygon. A regular pentagon has five lines of reflectional symmetry, and rotational symmetry of order 5 (through 72°, 144°, 216° and 288°). A convex pentagon has no angles pointing inwards. More precisely, no internal angles can be more than 180°. When any internal angle is greater than 180° it is concave.
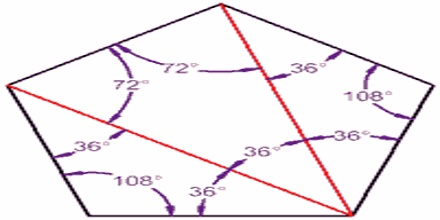
Properties: A regular pentagon has:
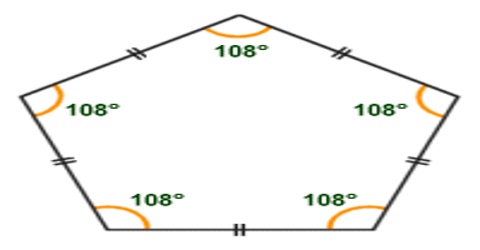
- Interior Angles of 108°
- Exterior Angles of 72°
- Area of approximately 1.7204774 × s2 (where, s = side length)
There are two types of pentagon on the basis of sides and angles:
- Regular pentagon
- Irregular pentagon
Regular pentagon: A pentagon which has all five sides equal in length and eventually, all the five angles are equal in measure, is known as regular pentagon. Each interior angle of a regular pentagon is 1080.
Irregular pentagon: An irregular pentagon is a pentagon which does not have all sides equal. Also, it does not have all angles equal.
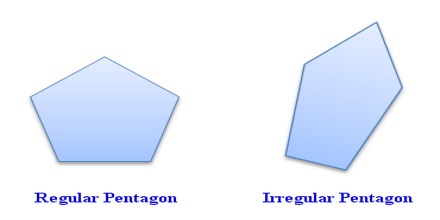
There are two types of pentagon on the basis of shape
- Convex pentagon
- Concave pentagon
Convex pentagon is a pentagon in which all five vertices are outwards. The pentagons demonstrated above are convex pentagons. A pentagon that has atleast one vertex pointing inwards is called a concave pentagon.