A nonparametric method is a mathematical approach to statistical inference that ignores the underlying assumptions about the form of the observed probability distribution. ANOVA, Pearson’s correlation, t-test, and other well-known statistical methods offer reliable information about the data being studied only if the underlying population follows those assumptions. It is a theory test that doesn’t need the populace’s dissemination to be portrayed by specific boundaries. Many hypothesis tests, for example, are predicated on the premise that the population follows a normal distribution with parameters μ and σ. Nonparametric tests, on the other hand, do not make this statement, making them useful when your data is strongly nonnormal and resistant to transformation.
Nonparametric statistics do not depend on the population data meeting the same assumptions as parametric statistics. The brought about estimation of covariate information is a typical issue in measurable information investigation because of the utilization of flawed proxy covariates, mistakes in factors, or the issue of missing information. Nonparametric measurements, along these lines, fall into a class of insights now and then alluded to as conveyance free. Where the population data has an uncertain distribution or the sample size is limited, nonparametric approaches are often used.
Nonparametric tests, on the other hand, are not completely free of assumptions about your results. For example, it’s critical to assume that the samples’ observations are independent and come from the same distribution. Likewise, in two-example plans the supposition of equivalent shape and spread is required. The nonparametric strategy helps in the demonstrating of suitable measurable strategies as a model structure apparatus in monetary time arrangement and econometrics. Although the nonparametric method is less efficient than the parametric approach, it does work under a few assumptions.
On various types of data, both parametric and nonparametric methods are commonly used. In most cases, interval or ratio data are required for parametric statistics. Nonparametric statistics, on the other hand, are usually used on data that are trivial or ordinal. Ostensible factors are factors for which the qualities have not quantitative worth. The nonparametric technique doesn’t need the populace under investigation to meet specific suppositions or explicit boundaries to describe the perceptions, similar to the case with parametric strategies. To provide an example, traditional parametric methods like the t-test and ANOVA only provide true and reliable results if the population under study meets certain assumptions.
Nonparametric tests have the following limitations:
- When the normality assumption is met, nonparametric tests are normally less efficient than parametric tests. If the data comes from a normal distribution, you are less likely to dismiss the null hypothesis when it is incorrect.
- Nonparametric experiments often necessitate the modification of hypotheses. Many nonparametric tests of the population center, for example, are tests of the median rather than the mean. If the population is not symmetric, the test does not answer the same question as to the corresponding parametric approach.
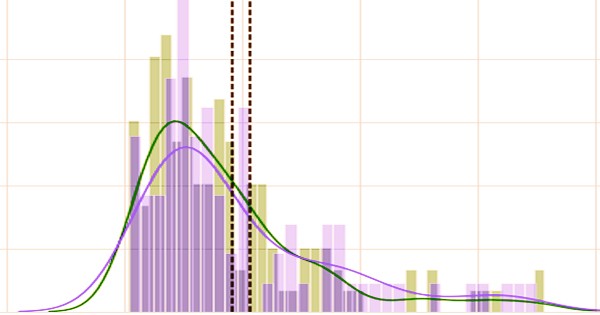
If a statistical approach follows the following criteria, it is considered nonparametric. First, the approach is applied to quantitative data where no population assumptions are made. Second, the strategy utilizes subjective information in a fairly casual manner; consequently, the nonparametric technique is an indicative instrument for a model structure where it tests, checks, appraises and approves information. Non-parametric methods have much broader applicability than parametric methods because they make less assumptions. They can be used in cases where little is understood about the application in question, for example. Non-parametric approaches are often more stable because they depend on fewer assumptions.
Albeit nonparametric measurements have the upside of meeting not many presumptions, they are less incredible than parametric insights. This implies that they may not show a connection between two factors when truth be told one exists. Another reason for using non-parametric methods is their simplicity. Non-parametric methods can be easier to use in some situations, even though parametric methods are justified. Due both to this straightforwardness and to their more prominent heartiness, non-parametric techniques are seen by certain analysts as leaving less space for ill-advised use and misconstruing.
Parametric and nonparametric approaches are used to analyze various types of data. Nonparametric data is concerned with nominal or ordinal data, while parametric data is concerned with interval or ratio data. The more extensive appropriateness and expanded strength of non-parametric tests includes some significant pitfalls: in situations where a parametric test would be proper, non-parametric tests have less force. To put it another way, a greater sample size might be needed to achieve the same level of confidence in conclusions.
Nonparametric methods are also widely used in financial econometrics to estimate returns, bond yields, volatility, return, and state price densities of stock prices. For instance, the technique is favored while looking at the additional time variety of stock costs and bonds. The term non-parametric isn’t intended to suggest that such models totally need boundaries yet that the number and nature of the boundaries are adaptable and not fixed ahead of time.
The curse of dimensionality problem has an effect on density estimation techniques. Using the nonparametric approach to estimate density function tends to be easy on the surface. Chi Square, Wilcoxon rank-sum test, Kruskal-Wallis test, and Spearman’s rank-order correlation are examples of nonparametric tests. A nonparametric strategy is hailed for its benefit of working under a couple of suspicions. Nonetheless, the idea is for the most part viewed as less amazing than the parametric methodology. In light of this, statisticians suggest using parametric methods in cases where both are acceptable.
Information Sources: