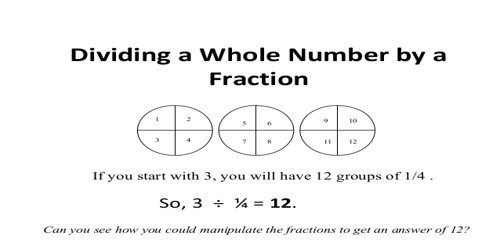
Dividing Fractions by Whole Numbers
There are two things to remember when dividing fractions. The first is that you can solve the problem by using the inverse operation. The inverse or opposite of division is multiplication. The second is that you will multiply by the reciprocal of the divisor. Remember that the reciprocal of a fraction is a fraction with the numerator and denominator change places.
To Divide Fractions by Whole Numbers:
- Treat the integer as a fraction (i.e. place it over the denominator 1)
- Invert (i.e. turn over) the denominator fraction and multiply the fractions
- Multiply the numerators of the fractions
- Multiply the denominators of the fractions
- Place the product of the numerators over the product of the denominators
- Simplify the Fraction
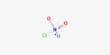
Example: Divide 2/9 by 2
- The integer divisor (2) can be considered to be a fraction (2/1)
- Invert the denominator fraction and multiply (2/9 ÷ 2/1 = 2/9 * 1/2)
- Multiply the numerators (2*1=2)
- Multiply the denominators (9*2=18)
- Place the product of the numerators over the product of the denominators (2/18)
- Simplify the Fraction if possible (2/18 = 1/9)
The Easy Way. After inverting, it is often simplest to “cancel” before doing the multiplication. Canceling is dividing one factor of the numerator and one factor of the denominator by the same number.
For example: 2/9 ÷ 2 = 2/9 ÷ 2/1 = 2/9*1/2 = (2*1)/(9*2) = (1*1)/(9*1) = 1/9
Information Source: