A team of scientists has produced irrefutable evidence that some spherical vortices exist in a stable condition. Mathematical models are essential for understanding the complicated behavior of atmospheric systems, and advances in this discipline can lead to better prediction models and better readiness for catastrophic weather occurrences.
Professor Kyudong Choi of UNIST’s Department of Mathematical Sciences has produced indisputable proof that certain spherical vortices exist in a stable condition, in a notable advance in the field of Mathematical Science. This ground-breaking discovery has far-reaching consequences for forecasting weather anomalies and enhancing weather prediction technology.
A vortex is a rapidly rotating patch of fluid, such as air or water, with extreme rotation. Typhoons and tornadoes are common examples, as shown in news stories. The mathematical argument provided by Professor Choi establishes the stability of specific forms of vortex configurations that can be encountered in real-world fluid flows.
Research on vortex stability has gained international attention. And it holds long-term potential for advancements in today’s weather forecasting technology.
Professor Choi
The research draws on Leonhard Euler’s 1757 Euler equation, which was developed to describe the flow of eddy currents. M. Hill, a British mathematician, established in 1894 that a ball-shaped vortex could maintain its shape indefinitely while rotating down its axis.
Professor Choi’s research confirms that Hill’s spherical vortex maximizes kinetic energy under certain conditions through the application of variational methods. By incorporating functional analysis and partial differential equation theory from mathematical analysis, this study extends previous investigations on two-dimensional fluid flows to encompass three-dimensional fluid dynamics with axial symmetry conditions.
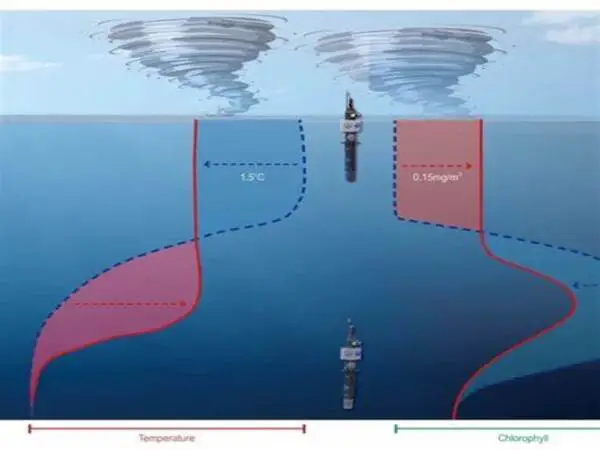
Hill noted one important feature: the existence of strong upward airflow at the front of the spherical vortex, which is common in phenomena such as typhoons and tornadoes. Professor Choi’s findings provide a foundation for future research utilizing residual time measurements associated with these ascending air currents.
“Research on vortex stability has gained international attention,” Professor Choi added. “[A]nd it holds long-term potential for advancements in today’s weather forecasting technology.”
This paper was published ahead of its formal release on July 24th via the online version of Communications on Pure and Applied Mathematics, thanks to support from the Korea Research Foundation under the Ministry of Science and ICT as well as UNIST.