In physics, the angular velocity specifies the angular speed at which an object is rotating along with the direction in which it is rotating. It applies to objects that move along a circular path. It is less common than linear velocity because it only applies to objects that are moving along a circular path. When an object is traveling along a circular path, the central angle corresponding to the object’s position on the circle is changing. It plays an eminent role in the rotational motion of an object.
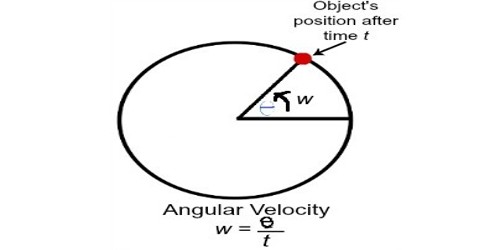
The angular velocity of an object is the object’s angular displacement with respect to time. It is a vector quantity. The SI unit of angular velocity is radians per second. But it may be measured in other units as well (such as degrees per second, degrees per hour, etc.). When it is measured in cycles or rotations per unit time (e.g. revolutions per minute), it is often called the rotational velocity and its magnitude the rotational speed. For example, a Ferris wheel may be rotating pi / 6 radians every minute. Therefore, the Ferris wheel’s angular velocity would be pi / 6 radians per minute.
Angular velocity is usually represented by the symbol omega (Ω or ω). It is measured in angle per unit time or radians per second (rad/s). The rate of change of angular velocity is angular acceleration. The direction of the angular velocity vector is perpendicular to the plane of rotation, in a direction which is usually specified by the right-hand rule. The angular displacement of in a given period of time gives the angular velocity of that object.
Mathematical Example: Calculate the angular velocity of a 0.300 m radius car tire when the car travels at 15.0m/s (about 54km/h ).
Strategy: Because the linear speed of the tire rim is the same as the speed of the car, we have v=15.0 m/s. The radius of the tire is given to be r=0.300 m. Knowing v and r, we can use the second relationship in v = rω, ω = v/r to calculate the angular velocity.
Solution
To calculate the angular velocity, we will use the following relationship:
ω=v/r.
Substituting the knowns,
ω = 15.0m/s0.300m = 50.0rad/s.